Question

A portion of $ 20,000 was invested at a 6% rate of return. The remainder was invested at a 4% rate of return. The total return on investment was $ 1000. Write a couple of equations that can be used to determine how much was invested at each rate.se invirtió una parte de $20,000 a una tasa de retorno del 6% El remanente se invirtió a una tasa de retorno del 4%. El retorno total de la inversión fue de $1000 escribe un par de ecuaciones que se puedan usar para allá cuanto se invirtió a cada tasa

Answer 1

• 0.06x+(800-0.04x)=1000

,

• The amount invested at both rates is $10,000.

Step-by-step explanation:

• Let the amount invested at a 6% rate of return = x

Since the total amount is $20,000

• The amount invested at a 4% rate of return = $(20,000-x)

Interest at 6%

`\begin{gathered} I=x*0.06*1 \\ I=0.06x \end{gathered}`

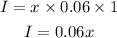
Interest at 4%

`\begin{gathered} I=0.04(20000-x) \\ I=800-0.04x \end{gathered}`

The total return on investment was $ 1000, therefore:

`\text{Total Interest = 0.06x+(800-0.04x)}=1000`

We can then solve for x to determine the amounts invested:

`\begin{gathered} 0.06x-0.04x=1000-800 \\ 0.02x=200 \\ x=(200)/(0.02) \\ x=10,000 \end{gathered}`

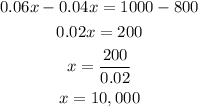
The amount invested at both rates is $10,000.