We are given the following function:
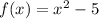
We are also given the following function:
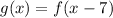
This means that the value of the function "g(x)" is obtained by substituting the value of "x" is "f(x)" for "x-7", like this:
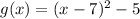
This type of transformation is a translation 7 units to the right, therefore, the graph of g(x) is shifted 7 units to the right of f(x).
The function is of the form:
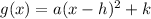
Where the vertex of the function is:
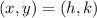
Therefore, the vertex of the function is:
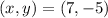
And the axis of symmetry is the x-coordinate of the vertex, therefore, the axis of symmetry is:
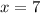
This means that the statements that apply is 2