This problem gives us several important pieces of information to keep in mind
1) A first-class plane ticket cost $880
2) A main-class plane ticket cost 65% less than the first-class ticket
Now let's solve each item
a)
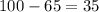
The cost of the main-cabin ticket is 35% of the cost of the first-class ticket
b)
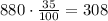
The price of the main-cabin ticket is $ 308
c)
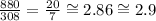
The first-class ticket costs 2.9 times the cost of the main-cabin ticket
d)
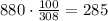
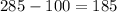
The first-class ticket costs 185% more than the main-cabin ticket.
C Explanation
We are asked to find how many times the first-class ticket costs compared to the main-class ticket, i.e. how many main-cabin tickets does the first-class ticket cost
So the first-class cost is to divide into the main-cabin cost, to find out how many times (how many main-cabin tickets) the first-class costs in comparison to the main-cabin
D Explanation
We already know the main-cabin and first-class ticket prices, the question refers to how much more a first-class ticket costs than a main-cabin ticket expressed as a percentage
For this, we take $308 as 100 percent so we want to know what percentage $880 corresponds to if we multiply by 100 and divide by 308, we find that the first-class ticket costs 285%, but let's remember that the question refers to how much more a first-class ticket costs than a main-cabin ticket
The first-class ticket costs 285% but if we subtract 100% from the main-cabin ticket this is the true percentage of how much more it costs
In conclusion, 285-100= 185%