Question

Below is the graph of . How would you describe the graph of ?A.g(x) stretches f(x) vertically by a factor of 2.B.g(x) compresses f(x) by a factor of 2.C.g(x) shifts f(x) up 2 units.D.g(x) shifts f(x) to the right 2 units.

Answer 1

Given: A graph of the function

Required: To describe the graph of the function

`g(x)=4ln(x)`

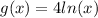
Explanation: Let c be a given non-zero real number. Then for a known function

`y=f(x)`

we have for a function

`g(x)=cf(x)`

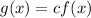
Then, if c>1 the graph g(x) stretches vertically and if 0The given function g(x) can be written as

`\begin{gathered} g(x)=4ln(x) \\ =2\cdot2ln(x) \\ =2f(x) \end{gathered}`

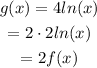
Hence, the given graph will vertically stretch by 2 units.

Final Answer: Option A is correct.