Question

Complete the square to findthe vertex of this parabola.x2 + 10x - y + 15 = 0([?], [ ]Enter

Answer 1

Answer;

`(-5,-10)`

Explanation;

Generally, we have the vertex form of a parabola as;

`y=a(x-h)^2+k`

Where, we have the vertex as (-h,k)

So, we proceed now to complete the square

We start by re-arranging and equating to zero as follows;

`y=x^2+10x+15`

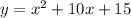
We divide the coefficient of x by 2, square it and add to both sides of the equation;

`\begin{gathered} x^2+10x+5^2=-15+5^2 \\ (x+5)^2=-15+25 \\ (x+5)^2=10 \\ (x+5)^2-10\text{ = y} \end{gathered}`

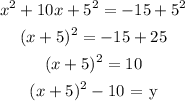
From the above, we have h as 5 and k as -10

The vertex which is (-h,k) would be (-5,-10)