Question

A line passes through the points A(-3,-2) and B(2,1). Does it also pass through the point C(5,3)?

Answer 1

`y=(3x)/(5)-(1)/(5)`

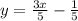
the line does not pass through the point C(5,3)

Step-by-step explanation

Step 1

find the equation of the line, to do that,

a) find the slope using:

`\text{slope}=(y_2-y_1)/(x_2-x_1)`

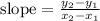
Let

`\begin{gathered} (x_1,y_1)=A(-3,-2) \\ (x_2,y_2)=B(2,1) \end{gathered}`

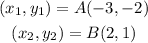
hence, the slope is

`\begin{gathered} \text{slope}=(1-(-2))/(2-(-3)) \\ \text{slope}=(3)/(5) \end{gathered}`

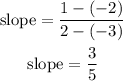
b) using the slope and the point A find the equation

`\begin{gathered} y-y_0=slope(x-x_0)\text{ Equation slope-point} \\ \text{replace} \\ y-(-2)=(3)/(5)(x-(-3)) \\ y+2=(3x)/(5)+(9)/(5) \\ y=(3x)/(5)+(9)/(5)-2 \\ y=(3x)/(5)-(1)/(5) \end{gathered}`

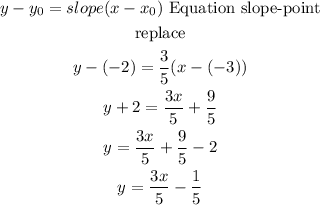
Step 2

Does it also pass through the point C(5,3)?

to answer this you have to replace the values for x and y and check if it is true

Let

C(5,3) x=5 y=3

`\begin{gathered} y=(3x)/(5)-(1)/(5) \\ 3=(3(5))/(5)-(1)/(5) \\ 3=(15)/(5)-(1)/(5) \\ 3=\frac{14}{5\text{ }}\text{ False} \\ \text{then} \end{gathered}`

the line does not pass through the point C(5,3)