Question

Determine if a triangle with the following sides is acute, obtuse, right, or cannot be determined.

Answer 1

According to the definition of triangle, the sum of 2 sides of a triangle is greater than the third side.

It means that for triangle ABC, it is true that:

`\begin{gathered} a+b>c \\ a+c>b \\ b+c>a \end{gathered}`

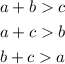
For the given value 3, 36 and 39, it is assumed that the sum of 3 and 36 must be greater than 39:

`\begin{gathered} 3+36>39 \\ 39>39 \\ 39=39 \end{gathered}`

The sum of 3 and 36 equals 39, is not greater than 39. It means the correct answer is Cannot be determined (Not a triangle)