Given that:
- The metal alloy is 15% copper and another alloy is 55% copper.
- The metalworker needs to create 50 kilograms of a 39% copper alloy.
Let be "x" the kilograms of metal alloy that is 15% copper and "y" the kilograms of metal alloy that is 55% copper.
Knowing that the metalworker needs to create a total of 50 kilograms, you can set up this first equation:
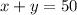
Knowing the percents that are given in the exercise, you can set up the second equation (remember that a percent can be converted to a decimal number by dividing it by 100):
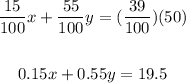
Now you can set up this System of Equations:
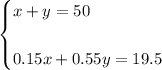
In order to solve it, you can use the Elimination Method:
1. Multiply the first equation by -0.15:
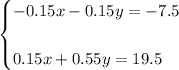
2. Add the equations:
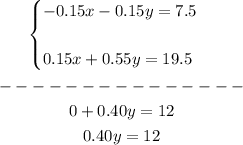
3. Solve for "y":
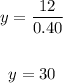
4. You can substitute the value of "y" into the first equation:
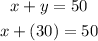
5. Solve for "x":

Hence, the answer is:
The metalworker should use 20 kilograms of the metal alloy that is 15% copper and 30 kilograms of the metal alloy that is 55% copper.