We can apply the Taylor expansion for the function:
![f(x)=\sqrt[]{x}](https://img.qammunity.org/2023/formulas/mathematics/college/eoshqhavec9ovuzgludbh20udpt0k7hgf6.png)
around x0=100.
The Taylor expansion with 2 terms (one differential) can be written as:
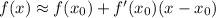
The value of f(x0) is 10.
We have to calculate the first derivative of f(x):
![f^(\prime)(x)=(d)/(dx)\lbrack x^{(1)/(2)}\rbrack=(1)/(2)x^{-(1)/(2)}=\frac{1}{2\sqrt[]{x}}](https://img.qammunity.org/2023/formulas/mathematics/college/63szd3ehzpxvnewogvec41p5613lu8novt.png)
Now, we can calculate f'(x0):
![f^(\prime)(x_0)=f^(\prime)(100)=\frac{1}{2\sqrt[]{100}}=(1)/(2\cdot10)=(1)/(20)](https://img.qammunity.org/2023/formulas/mathematics/college/ycogu3cec7kvaciqhvtcfnrsgxd9i3wdo6.png)
We then can calculate:
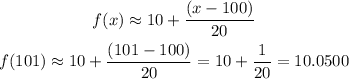
Answer: 10.0500.