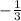Given the points:
(x1, y1) ==> (3, 4)
(x2, y2) ==> (6, 3)
Let's find the slope of the line passing through the points.
To find the slope, apply the slope formula:

Plug in the values and solve for the slope, m:
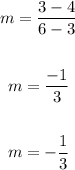
Therefore, the slope of the line passing through the points is:
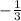
ANSWER: