Question

You play a simple game with the 26 letters of the alphabet. If a vowel comes up you pay Harry $5. If a consonant (not a vowel) comes up, Harry pays you $1.50. If you play this game 100 times, how much do you expect to win or lose?Answer:

Answer 1

From the statement, we know that:

• you play a game with 26 letters of the alphabet,

,

• if a vowel comes up, you pay harry $5,

,

• if a consonant comes up, you win $1.50.

We define:

• Event 1 = a vowel comes up → you earn ,E_1 = -$5,,

,

• Event 2 = a consonant comes up → you earn ,E_2 = $1.50,.

The mean earned money is given by the formula:

`\bar{E}=p_1\cdot E_1+p_2\cdot E_2\text{.}`

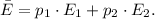
Where:

• p_1 ,= probability of Event 1 = # of vowels / 26 = ,5/26,,

,

• p_2, = probability of Event 2 = # of consonants / 26 = ,21/26,.

Replacing the data in the formula for the mean earned money, we get:

`\bar{E}=(5)/(26)\cdot\text{ (-\$5)}+(21)/(26)\cdot\text{ \$1.50 }=\text{ \$0.25.}`

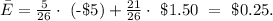
Answer

You expect to win a mean of $0.25.