Answer:

Explanation:
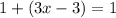
First, you have to take the one that is outside of the Parenthesis and take it to the other side. That would mean it would be this:
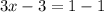
1 - 1 is 0
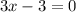
Move the -3 to the other side, which makes it positive;
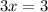
Divide both sides by 3

If you want to check it, you can replace 1 in the original equation:
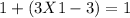
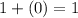
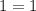
So x=1