Given:
The price of 3 citrons and 4 fragrant wood apples is 36 units.
The price of 4 citrons and 3 fragrant wood apples is 41 units.
To find: The price of citron and the price of fragrant wood apple?
Step-by-step explanation:
Let,
The price of citrons = x
The price of fragrant wood apple = y
We can write an equation as
The price of 3 citrons and 4 fragrant wood apples is 36 units.

and
The price of 4 citrons and 3 fragrant wood apples is 41 units.
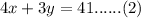
Now, Here we use the elimination method,
So, multiply by 4 in Eq.1 and multiply by 3 in Eq. 2
We get,
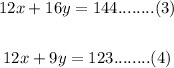
Now, subract Eq.4 from Eq.3
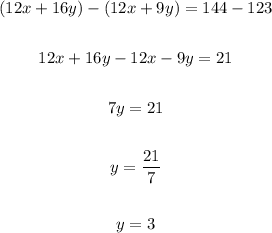
Put y = 3 in Eq.1
We get,
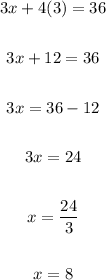
Hence, x = 8 and y = 3
Therefore,
The price of citrons = x = 8 units
The price of fragrant wood apple = y = 3 units
Answer: The price of citrons is 8 units and the price of fragrant wood apple is 3 units.