Concept
The process is a combination which is the selection of r objects out of n objects.
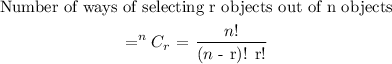
Next,
Given data, you are to select 3 members out of 7 members.
r = 3
n = 7
Substituting n and r into the equation we get a number of ways.

Next, use your calculator to 7! , 4! and 3!.
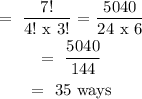
Final answer = 35 ways