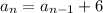Question

HELP!!!!!!!!!!!!!!!!!!!

Answer 1

4) a₁₅ = 128

5) B.
`a_n=a_(n-1)+6`

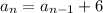
Explanation:

4)

This is an arithmetic sequence, where all terms have a common difference:

`a_n=a_1+(n-1)d`

Here, the common difference is 9.

`11-2=9\\20-11=9`

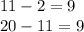
Use that to write the equation and solve for a₁₅:

`a_n=2+(n-1)9\\\\a_(15)=2+(15-1)9\\a_(15)=2+(14)9\\a_(15)=2+126\\a_(15)=128`

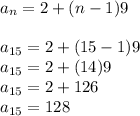
5)

This is also an arithmetic sequence, but it is a recursive arithmetic sequence in the form of:

`a_n=a_(n-1)+d`

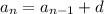
This simply states that each term is d more than the term before it.

Here, the common difference is 6:

`2--4=6\\8-2=6\\14-8=6`

And the equation for this is B.
`a_n=a_(n-1)+6`