ANSWER
u = 12.37(0.242, -0.970)
v = 20.12(-0.89, 0.45)
Step-by-step explanation
We want to write u and v in trigonometric form.
1. for u
Determine the component form of u
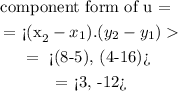
Determine the magnitude of the vector u
![\begin{gathered} \mleft\Vert u\mleft\Vert\text{ =}\sqrt[]{3^2+(-12)^2}\mright?\mright? \\ \text{ = }\sqrt[]{9+144} \\ \text{ = }\sqrt[]{153} \\ \mleft\Vert u\mleft\Vert\text{ = 12.37}\mright?\mright? \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/1qdy4wl47zh1v85dvg6gibal5vwokfj6yn.png)
Determine the angle theta
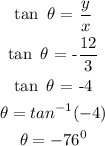
But this is in the wrong quadrant
so,
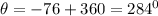
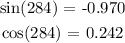
Hence, writing u in trigonometric form:
u = 12.37(0.242, -0.970).
2. for v
Determine the component form of v
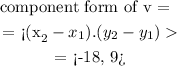
Determine the magnitude of the vector v
![\begin{gathered} \Vert v\Vert\text{ =}\sqrt[]{(-18)^2+(9)^2} \\ \text{ = }\sqrt[]{405} \\ \text{ = 20.12} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/u7lkqoz4ybyv5h9nydrq0w7we90k5vbknj.png)
Determine the angle theta
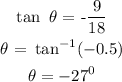
But this is in the wrong quadrant
so,

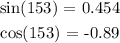
Hence, writing u in trigonometric form:
v = 20.12(-0.89, 0.45)