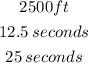
1) Let's find the maximum point of this quadratic equation, by using the following formula to get the h coordinate of the vertex and then plug into the function the value we found.
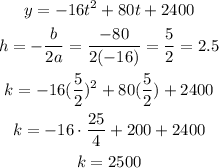
So the maximum height is given y the point at the vertex (2.5,2500) .i.e. 2500 feet
2) From the previous part, we can tell It takes 12.5 seconds for the rocket to get 2500 ft high.
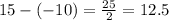
We are considering the rocket to strt flying off at x=-10.
3) To find when the rocket reaches the ground is to find the roots of this quadratic equation, and then pick one of them. So let's do it:
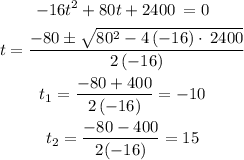
Since we cannot deal with the negative measurement of time. We can tell that
Note that the question is after it is fired consider the rocket is fired at x=-15 and hits its peak 2.5 seconds so the rocket hits the ground 25 seconds after it was launched at t=1