Given:
Speed of train 1 = 60 mph due west (leaves at noon).
Speed of train 2 = 70 mph (leaves at 1 pm).
Let's find how far apart they will be on a straigth line distance at 4 p.m.
We have:
• Time taken by train 1 = 4:00 - 12:00 = 4 hours
,
• Time taken by train 2 = 4:00 - 1:00 = 3 hours
Apply the formula:
Distance = speed x time
Thus, we have:
• Distance covered by train 1 = 60 x 4 = 240 miles
,
• Distance covered by train 2 = 70 x 3 = 310 miles
Now, to find how far apart, apply Pythagorean Theorem:
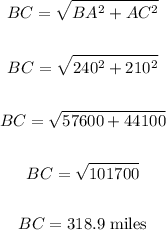
Therefore, the trains will be 318.9 miles apart on a straight line distance travelling due north.
• ANSWER:
318.9 miles