Question

This equation is LINEAR. Which equation would matches the table?

Answer 1

Given: A table of values representing a linear equation (relationship)

Required: To get the equation of the relationship.

Generally, the equation of a linear relationship takes the form

`\begin{gathered} y\text{ = ax + b} \\ \text{where a is the gradient (or slope)} \\ \text{and b is the y-intercept} \end{gathered}`

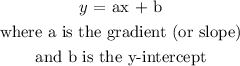
First, let us get b (i.e. the y-intercept). This is the value of y when x= 0.

From the table, this is 20. (0,20)

Hence, the equation will be

`y=\text{ ax + 20}`

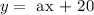
Next we find the gradient (slope), a.

We do this by testing the equation above with any other value of x except 1.

We will be taking the point (1, 17) from the table

`\begin{gathered} y=ax\text{ + 20. Point (1,17)} \\ 17\text{ = a(1) + 20} \\ 17\text{ = a + 20} \\ a=\text{ 17 - 20} \\ a=\text{ -3} \end{gathered}`

Final answer:

Hence the equation formed will be:

y= -3x + 20. Option (D)