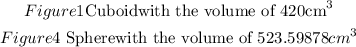Volume of figure 1
The figure is a Cuboid
The formula for the volume of a cuboid is,
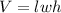
Given:
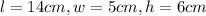
Therefore,
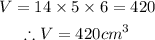
Volume of figure 2
The figure is a Cylinder.
The formula for the volume of a cylinder is,

Given:
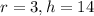
Therefore,
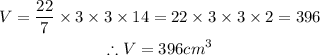
Volume of figure 3
The figure is a Cone
The formula for the volume of a cone is,
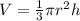
Given:
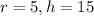
Therefore,
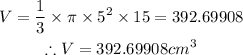
Volume of figure 4
The figure is a Sphere
The formula for the volume of a sphere is,
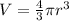
Given:
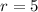
Therefore,
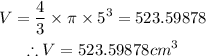
Volume of figure 5
The figure is a Rectangle-based pyramid
The formula for the volume of a rectangle-based pyramid is,
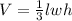
Given:
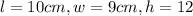
Therefore,
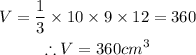
Hence, the figures with a volume greater than 400 cubic centimeters are