We have to make the conversations
mass rod=100g=.1 kg
mass =50g=0.05 kg
in order to obtain the weight, we need to multiply the mass by the gravity in this case 9.8 m/s^2
w_rod= .1*9.8=0.98 N
w_mass=.05*9.8=0.049N
Then wee need to draw a diagram
We have RA and RB the reactions of the support
First we need to find the sum of forces
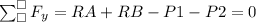
Then we analyze the moments of force
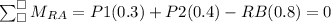
then we can calculate RB
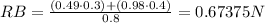
then we can calculate RA
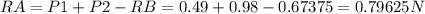
the reactions will be
RA=0.79625N=0.80N
RB=0.67375N=0.67N