ANSWERS
a. 4 m/s
b. 50 s
c.
d. 6 m/s
e. 4 m/s
Step-by-step explanation
a. The speed of each runner at a certain time is the slope of the graph at that time. Because both position vs time graphs are linear, the slope is constant and thus, the speed is the same at all times.
Let's find the slope of the graph of runner B. We can use the formula for the slope:
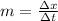
For runner B Δx and Δt are,
So the slope is,
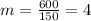
And the speed of runner B is 4 m/s at all times.
b. Since the graph of the position of runner A is linear and starts at (0, 0) we know that the position has the equation,
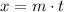
Where m is the slope - which represents the speed of the runner,
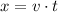
As before, we can find the speed with the slope formula. In this case Δx = 600 and Δt = 100,
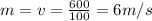
The equation for the position of runner A is,
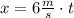
Solving for t,
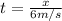
If runner A run 300 meters,
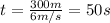
He has run for 50 seconds.
c. As stated before, the speed of the runners is represented by the slope of the graph. If we want slower speed we need a smaller slope, so any of these lines - and the lines in-between, can represent the position of a runner with slower speed than runners A and B,
I'll choose any of these and show it in the ANSWER section.
d. The speed of runner A is 6m/s. See item b for explanation.
e. The speed of runner B is 4 m/s. See item a for explanation.