By definition, a geometric progression, also known as a geometric sequence, is a sequence of non-zero numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio.
The terms of a geometric sequence are given by
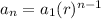
Where r represents the common ratio and a1 represents the first term.
The sequences may increase, decrease, or alternate between increasing and decreasing(it all depends on the value of the common ratio).
If the first term is zero, then all terms are zero—not a very interesting sequence. Since division by zero is undefined, the common ration of such a sequence would be undefined.
There are some sequences that have a common difference between particular pairs of terms. However, a sequence that has the same difference between all adjacent pairs of terms is called an arithmetic sequence, not a geometric sequence.
Any sequence has terms numbered by the counting numbers: term 1, term 2, term 3, and so on. Hence the domain is those natural numbers. The relation describing a geometric sequence is an exponential relation. It can be evaluated for values of the independent variable that are not natural numbers, but now we're talking exponential function, not geometric sequence.
From those statements, those that correctly describe a geometric sequence are the first two statements.
Geometric sequences have a common ratio between terms.
Geometric sequences are restricted to the domain of natural numbers.