Given the inequality:

To find the solution set, the goal is to get the n alone between the inequality signs. First we have to get rid of the -12. For this, we can add 12 on both sides of the inequality to get the following:
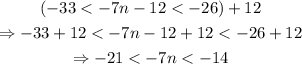
Notice that -7 is multiplying n. To get rid of it, we have to divide by -7 the whole expression and since we are doing this with a negative number, then the inequality signs are going to change:
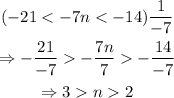
therefore, the solution is (2,3) and it looks like this in the number line: