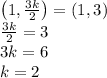Answer:
k = 2
Explanation:
We'll need the Midpoint Formula: the midpoint of a segment joining points
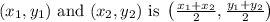 . In other words, average the x's, then average the y's.
. In other words, average the x's, then average the y's.
The midpoint of a diameter is the centre of the circle!
The midpoint of the segment joining (-3, 2k) and (5, k) is
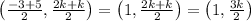
That last expression is the centre of the circle; it is the same as (1, 3).