The given subraction is:

The LCD is the LCM of the denominator. so the LCD is found as follows:
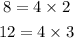
LCM is common multiplied by the uncommon factors of the two denominators so the LCD is:

The fraction is solved as follows:

Hence the LCD is 24 and the answer is option E which is 5/24.