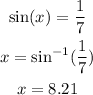We have to solve the equation:
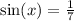
To solve for an angle of a trig ratio, we take the inverse trig of the "constant" to the other side. That will let us solve for the "angle".
Thus, we can take the inverse sine of 1/7th to get the value of the angle "x".
Shown below: