Given,
The weight of the child, W=500 N
The weight of the friend, w= 300 N
The distance from the pivot to the child, D=2.0 m
The distance from the pivot to the friend, d=2.0 m
a.
The moment of the child is given by,
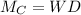
On substituting the known values,
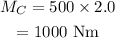
Thus the moment of the child is 1000 Nm
b.
The moment of the friend can be calculated using the formula,
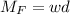
On substituting the known values,

Thus the moment of the friend's weight is 600 Nm
c.
To balance the seesaw the total moment to the right of the center must be equal to the total moment to the left of the center.
The distance of the right end from the center of the seesaw is s=2.5 m
Thus,
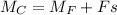
Where F is the downward force needed.
Thus the downward force needed to be applied at the right end is given by,
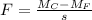
On substituting the known values,
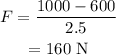
Thus the downward force that must be applied at the right-hand end of the seesaw is 160 N.