Equation of a Circle
The equation of a circle with center at (h,k) and radius r is:

We know the endpoints of a diameter of a circle are (1,3) and (-3,1). The center of the circle is located at the midpoint of the segment.
Let's find the coordinates of the midpoint:
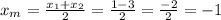
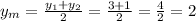
The center is located at (-1,2)
The radius is the distance from the center to any of the endpoints. Let's calculate that distance:
![r=\sqrt[]{(-1-1)^2+(2-3)^2}=\sqrt[]{4+1}=\sqrt[]{5}](https://img.qammunity.org/2023/formulas/mathematics/college/rn1ufhmycz2lup9v6174hv3d07590q2dtg.png)
Thus, the equation of the circle is:
![\begin{gathered} (x+1)^2+(y-2)^2=(\sqrt[]{5})^2 \\ \text{Operating:} \\ (x+1)^2+(y-2)^2=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/vpapmca2ytqucp9llauu2g5xo5e44upioi.png)
Choice C.