Let x be the profit of a bracelet and y the profit of a necklace.
Yesterday she sold: 9 bracelets and 3 necklaces, for a profit of $144:
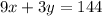
Today, she made a profit of $279 by selling 10 bracelets and 9 necklaces:
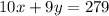
System of equations:
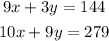
To solve by elimination:
1. Multiply the first equation by -3:
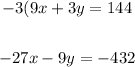
2. Add equation you get in step 1 and the second equation in the system:
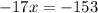
3. Use the equation you get in step 2 to solve x:
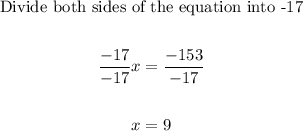
4. Use the value of x to solve y:
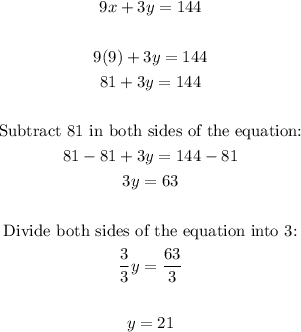
Then, the solution for the system is :
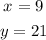
Kaylee earns a profit of $9 from every bracelet and $21 from every necklace