Question

Algebra 14.11 Solve a system of equations using elimination: word problems NHRYou have prizes to reveal! GotWrite a system of equations to describe the situation below, solve using elimination, and fill inthe blanksThe administrative assistant at a software company often provides breakfast when there is amorning meeting. For last week's sales meeting, she purchased 6 dozen doughnuts and 2dozen croissants, spending a total of $42. In preparation for yesterday's safety meeting, shespent $40 on 2 dozen doughnuts and 5 dozen croissants. Assuming she purchased the itemsat the same bakery both times, how much does a dozen of each cost?A dozen doughnuts costs $and a dozen croissants costs $Submit

Answer 1

Define a variable for the unkwons

`\begin{gathered} \text{Let } \\ A\text{ dozen of doughnuts cost=\$x} \\ A\text{ dozen of Croissant cost=\$y} \end{gathered}`

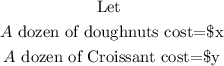
Then

6 dozen of doughnuts and 2 dozen of croissant cost $42, is written as

`6x+2y=42\ldots\text{equation 1}`

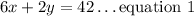
Similarly

$40 for 2 dozen of doughnuts and 5 dozen of croissant is witten as

`2x+5y=40\ldots\text{equation 2}`

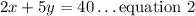
Applying Elimination to solve the two system of equation, we have

`\begin{gathered} 6x+2y=42\ldots\text{equation 1} \\ 2x+5y=40\ldots\text{equation 2} \\ To\text{ eliminate x multiply equation 2 by 3 and equation 1 by 1} \\ 1*(6x+2y=42)\rightarrow6x+2y=42 \\ 3*(2x+5y=40)\rightarrow6x+15y=120 \end{gathered}`

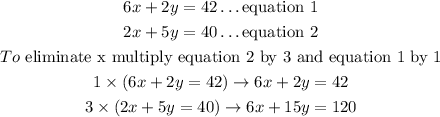
Then, subtract the equation obtained above

`\begin{gathered} 6x+2y=42 \\ 6x+15y=120 \\ -13y=-78 \\ \text{Divide both sides by -13} \\ -(13y)/(-13)=-(78)/(-13) \\ \\ y=6 \end{gathered}`

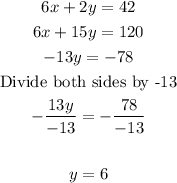
Hence Y=6

Then you Eliminate Y from eqaution 1 an d 2 by

Multiplying equation 1 by 5 and equation 2 by 2

`\begin{gathered} 5*(6x+2y=42)\rightarrow30x+10y=210 \\ 2*(2x+5y=40)\rightarrow4x+10y=80 \end{gathered}`

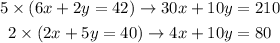
The sunbtract the equation obtained

`\begin{gathered} 30x+10y=210 \\ 4x+10y=80 \\ 26x=130 \\ \text{Divide both sides by 26} \\ (26x)/(26)=(130)/(26) \\ \\ x=5 \end{gathered}`

Hence X=5

Therefore

A Dozen of doughnuts cost $5

A Dozen of Croisant cost $6