The maximum and minimum points of a function happens at the zeros of the first derivative. The power rule is
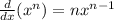
Using this property in our function, we're going to have
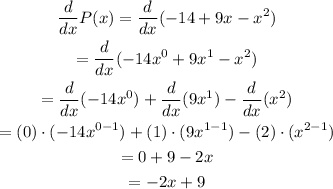
which is a linear function, with a zero at
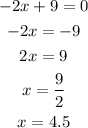
x = 4.5, therefore, 4500 Boombotix speakers should be sold to maximize the profit.