Question

Is this a direct variation or not? And explain how you know

Answer 1

The form of the direct variation is

`y=kx`

Where k is the constant of variation

Since the given figure is a parabola that represents a quadratic equation, then

The equation should be

`y=kx^2`

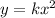
Let us use the points on the graph to check if all values of k will be equal

If they are equal, then it is a direct variation,

if they are not equal, then it is not a direct variation

Let us use points (2, 2), (4, 8), and (-3, 4.5)

`\begin{gathered} x=2,y=2 \\ 2=k(2)^2 \\ 2=4k \\ (2)/(4)=(4k)/(4) \\ (1)/(2)=k \end{gathered}`
`\begin{gathered} x=4,y=8 \\ 8=k(4)^2 \\ 8=16k \\ (8)/(16)=(16k)/(16) \\ (1)/(2)=k \end{gathered}`

`\begin{gathered} x=-3,y=4.5 \\ 4.5=k(-3)^2 \\ 4.5=9k \\ (4.5)/(9)=(9k)/(9) \\ (1)/(2)=k \end{gathered}`

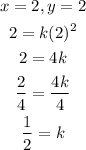
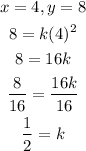
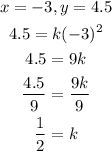
Since the values of k are equal, then it is a direct variation

Yes, it is a direct variation