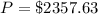Question

Use the continuous compound interest formula to find the indicated valueA = $5,200; r = 7.91%; t = 10 years; P = ?Round to two decimal places as needed.)(

Answer 1

To answer this question, we have to use the next formula, which is the continuous compounding interest formula:

`A=Pe^(rt)_{}`

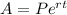
Where:

• A is the accrued amount. In this case, we have A = $5,200.

,

• P is the principal. This is the amount we need to find.

,

• r is the interest rate. In this case, the value is r = 7.91% or 7.91/100.

,

• t is time in years. In this case, t = 10 years.

,

• e is the "e" number (e is approximately 2.71828182846)

Therefore, we have:

`\begin{gathered} A=Pe^(rt)_{} \\ 5200=Pe^{(7.91)/(100)\cdot10} \end{gathered}`

Now, we have to solve the equation by using the inverse function of the natural exponential function, namely, the natural logarithm as follows:

`\begin{gathered} 5200=Pe^{7.91\cdot(10)/(100)} \\ 5200=Pe^{7.91\cdot(1)/(10)} \\ 5200=Pe^{(7.91)/(10)}=Pe^(0.791) \\ \end{gathered}`

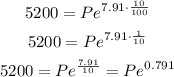
Now, we can see that is not necessary to use the natural logarithm. We have to divide both sides by the resulting exponential value:

`\begin{gathered} (5200)/(e^(0.791))=P(e^(0.791))/(e^(0.791))\Rightarrow(e^(0.791))/(e^(0.791))=1,(a)/(a)=1 \\ \end{gathered}`

Therefore, we finally have:

`P=(5200)/(e^(0.791))=2357.63412214`

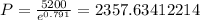
If we round the resulting value to two decimal places, we have that:

`P=\$2357.63`