Question

Noah thinks the answers to these two questions will be the same. This year, a herd of bison had a 10% increase in population. If there were 550 bison in the herd last year, how many are in the herd this year?This year, another herd of bison had a 10% decrease in population. If there are 550 bison in the herd this year, how many bison were there last year?1. Do you agree with Noah? Select your choice from the drop down menu. 2. Explain your reasoning.Type your response in the space below.

Answer 1

The answer to these two questions are not the same. We don't agree with Noah.

Reason:

Let's start with the first question.

1. Given: rate = 10% increase, base/original number = 550 bison

10% of 550 is:

`.10*550bison=55bison`

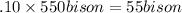
10% of 550 is 55. Hence, there is an increase of 55 bison this year. This year, there are 550 + 55 = 605 bison in the herd.

2. Given: rate = 10% decrease, percentage/final number = 550 bison

Applying the concept of Percentage = Base x Rate, we can get the Base or the original number of bison before the decrease by dividing Percentage over Rate.

`\text{Base}=\frac{\text{Percentage}}{\text{Rate}}`

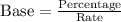
Filling in the formula with the given values in question 2, we have:

`\text{Base}=(550)/(1-.10)=(550)/(.90)=611.11\approx611bison`

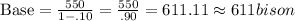
Last year, there were 611 bison.

As we can see, the answer for number 1 is 605 bison while the answer for the number 2 is 611 bison. The answer of the two questions are not the same.