ANSWER
2
Step-by-step explanation
We have the equation:
x + 2y = 2
To find the slope of the line perpendicular to the line given, we have to first write the equation in slope intercept form.
That is in the form:
y = mx + c
where m = slope
c = intercept
=> 2y = 2 - x
y = (-1/2)x + 1
The slope of this line is -1/2.
Now, to find the slope of the line perpendicular to it, we have to find the negative inverse of the slope.
That is:
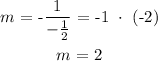
That is the slope of the line perpendicular to the line given.