Question

What percentage of customers did not have a smart phone

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Draw the given table

STEP 2: Explain how to get the relative frequency

The relative frequency is derived by dividing the columns by the total frequency.

STEP 3: Complete the table

`\begin{gathered} \text{Total for Row 2} \\ \Rightarrow82+47=129 \\ \\ \text{The number of No Text Message Plan/Has Smart Phone} \\ 46-13=33 \\ \\ \text{The number of Total for Does Not have Smartphone} \\ 47+13=60 \end{gathered}`

STEP 4; Draw the complete table

STEP 5: Calculate the relative Frequency

`\begin{gathered} \text{Text Messaging Plan/Has Smart Phone} \\ (82)/(175)=0.46857 \\ \\ \text{No Text Messaging Plan/Has Smart Phone} \\ (33)/(175)=0.18857 \\ \\ \text{Text Messaging Plan/Does Not Have Smart Phone} \\ (47)/(175)=0.26857 \\ \\ \text{No Text Messaging Plan/Does Not Have Smart Phone} \\ (13)/(1750)=0.0742857 \end{gathered}`

STEP 6: Calculate the percentage of customers without smartphones

`\begin{gathered} (60)/(175)*100=(6000)/(175)=34.28571429 \\ \approx34\% \end{gathered}`

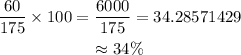
Hence, the percentage of customers without smartphones is 34%