Ok, so
The point slope form of the line is given by the following formula:
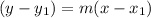
Where
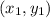
Is a point of the line, and m is the slope.
If we replace our values:
Slope = 7
Point = (-6, 1)
We obtain that the equation is:
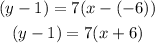
To find the slope intercept form of the equation, we distribute in the brackets:

And the equation of our line in the slope intercept form will be:
y=7x+43