Given:

To find:
The interval at which the function is increasing, decreasing and constant.
Step-by-step explanation:
We know that,
For a function, y = F(x), if the value of y is increasing on increasing the value of x, then the function is known as an increasing function.
For a function, y = F(x), if the value of y is decreasing on increasing the value of x, then the function is known as a decreasing function.
According to the graph,
The function is increasing in the interval,
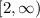
Because, if x increases from 2, the value of y increases.
The function is decreasing in the interval,
![(-\infty,-2]](https://img.qammunity.org/2023/formulas/mathematics/college/9mwercsdyqe76rueqcuq377k2073sx4ow3.png)
Because, if x increases from negative infinity, the value of y decreases.
As we know, a constant function is a function whose output value is the same for every input value.
Here, the function is not constant at any of the intervals.
Final answer:
Increasing:
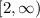
Decreasing:
![(-\infty,-2]](https://img.qammunity.org/2023/formulas/mathematics/college/9mwercsdyqe76rueqcuq377k2073sx4ow3.png)
Constant: DNE.