Question

What are the dimensions of the rectangle with Maximum area?

Answer 1

Let x be the length of the rectangle and y be the width of the rectangle, then we can set the following equations:

`\begin{gathered} 2x+2y=40, \\ A=x\cdot y\text{.} \end{gathered}`

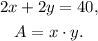
Solving the first equation for x we get:

`\begin{gathered} 2x=40-2y, \\ x=(40)/(2)-(2y)/(2), \\ x=20-y\text{.} \end{gathered}`

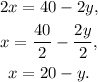
Substituting x=20-y in the second equation we get:

`\begin{gathered} A=(20-y)* y, \\ A=20y-y^2. \end{gathered}`

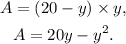
Now, we will use the first and second derivative criteria to find the maximum.

The first and second derivatives are:

`\begin{gathered} A^(\prime)(y)=20-2y. \\ A^(\prime\prime)(y)=-2. \end{gathered}`

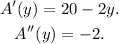
Since the second derivative is a negative number that means that A(y) reaches a maximum when A´(y)=0.

Solving A´(y)=0 for y we get:

`\begin{gathered} 20-2y=0, \\ 20=2y, \\ 10=y\text{.} \end{gathered}`

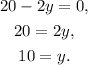
Now, substituting y=10 in x=20-y, we get:

`x=20-10=10.`

Answer:

Length 10 yards.

Width 10 yards.