Question

How long will it take for a $4000 investment to grow to $5780 at an annual rate of 4%, compounded quarterly? Assume that no withdrawals are made. Do not round any intermediate computations, and round your answer to the nearest hundredths.

Answer 1

The working equation when dealing with problems regarding compounded interest is

`A=P(1+(r)/(n))^(nt)`

where A is the future value, P is the principal value, r is the annual rate, and n is the number of compounding periods.

The problem compounds quarterly, hence, we have n = 4.

We derive the working equation to solve for t, as follows:

`\begin{gathered} (A)/(P)=(1+(r)/(n))^(nt) \\ \ln ((A)/(P))=\ln ((1+(r)/(n))^(nt)) \\ nt\ln ((1+(r)/(n)))=\ln ((A)/(P)) \\ t=(\ln ((A)/(P)))/(n\ln ((1+(r)/(n)))) \end{gathered}`

Substitute the values of A, P, n, and r on the derived equation above and solve for t, we get

`\begin{gathered} t=(\ln ((5780)/(4000)))/(4(\ln (1+(0.04)/(4)))) \\ t=(\ln (1.445))/(4(\ln (1.01))) \\ t=(0.368)/(4(0.00995)) \\ t\approx9.25 \end{gathered}`

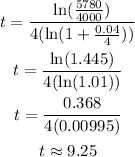
Therefore, the $4000 investment grows to $5780 in 9.25 years.