To obtain the probabilities asked about in the question, we proceed as follows:
Step 1: Recall the definition of the probability of any event E occuring, which is as follows:
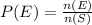
where:
n(E) = number of elements in set E
n(S) = number of elements in the entire sample space
Step 2: Identify the data given in the question, as follows:
We have that:
Total sample = 500 people
Tongue twisters = 225
Step 3: Interpret the question prompts, as follows:
a) Find the probability that both of the two people selected from the sample can twist their tongues.
To do this, we write out the probabilities associated with the first and second selection of such two people from the sample, as below:
- the probability associated with the first selection is:
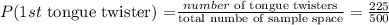
- the probability associated with the second selection is:
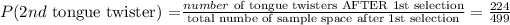
Now, the the probability that both of the two people selected from the sample can twist their tongues is therefore:
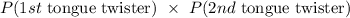
Thus:
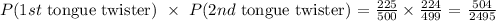
The answer is thus : 504/2495
b) Find the probability that both of the two people selected from the sample can NOT twist their tongues
To do this, we write out the probabilities associated with the first and second selection of such two people from the sample, as below:
- the probability associated with the first selection is:

- the probability associated with the second selection is:
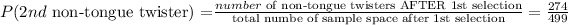
Now, the the probability that both of the two people selected from the sample can NOT twist their tongues is therefore:
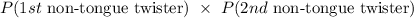
Therefore:

The answer is thus : 1507/4990