Question

10 9 8 7 6 5 4 19 -10 -9 -8 -7 X -6 -5 -4 -3.-2 4 5 6 7 8 9 10 3 756789 -10 Slope of one of the dotted lines: Slope of the reflection line:what is the slope of the reflection line

Answer 1

The slope of one of the dotted lines: -1

The slope of the reflection line: 1

Step-by-step explanation:

The slope of a line that passes through two points (x1, y1) and (x2, y2) can be calculated as:

`m=\frac{\text{Rise}}{\text{Run}}=(y_2-y_1)/(x_2-x_1)`

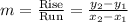
Therefore, the slope of the dotted line that passes through the points (-1, 2) and (5, -4) is:

`m=(-4-2)/(5-(-1))=(-6)/(5+1)=-(6)/(6)=-1`

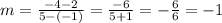
So, the slope of one of the dotted lines is -1

In the same, we can calculate the slope of the reflection line, replacing (x1, y1) by (2, -1) and (x2, y2) by (0, -3), so:

`m=(-3-(-1))/(0-2)=(-3+1)/(-2)=(-2)/(-2)=1`

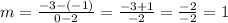
So, the slope of the reflection line is 1.