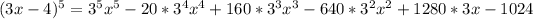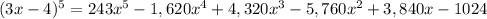Answer:
Explanation:
We worry about 2 things:
-terms power
-coefficient for each term
(3x-4)^5, has 2 terms 3x and -4
-Start with the first term to the highest power 5 and second term to the lowest power 0, then the high power goes down and low power increases until the first term has the lowest power 0 and the second term has the highest power 5.
-The coefficients for each term we take it from the Pascal triangle.
For the the power 5 the coefficients are 1, 5, 10, 10, 5, 1
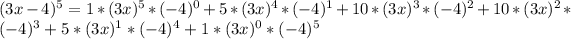
Simplify: