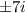You have the following expression:
![\pm\sqrt[]{-49}](https://img.qammunity.org/2023/formulas/mathematics/high-school/pqra7vq0q50xmxx6hev8j3rge743th5mzw.png)
In order to express the previous number as an expression with the imaginary unit, you consider the following:
![\begin{gathered} 49=7^2 \\ \sqrt[]{-1}=i \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/q6p0swdyrpe525q2yifl0luzxdb7ieomzo.png)
where the square root of -1 is the imaginary unit i by definition. Thus, by replacing you obtain:
![\pm\sqrt[]{-49}=\pm\sqrt[]{(-1)(49)}=\pm\sqrt[]{(-1)(7^2)}=\pm7\sqrt[]{-1}=\pm7i](https://img.qammunity.org/2023/formulas/mathematics/high-school/4cdv4d7e08nokmbj869v113kb8xhhd9en9.png)
Hence, the given expression in terms of the imaginary unit is :