Question

You are given a bag that contains 7 red, 8 blue, 2 orange, 3 white, and 6 green marbles.1) What is the probability of selecting a red marble replacing it and then selecting a green marble?2) What is the probability of selecting a blue marble replacing it and then selecting another blue marble?3) What is the probability of selecting a white marble and then a green marble without replacing the first marble?4) What is the probability of selecting a red marble and then selecting another red marble without replacing the first marble?

Answer 1

replacementThe marbles given are

`\begin{gathered} \text{RED}=7 \\ \text{BLUE}=8 \\ \text{ORANGE}=2 \\ \text{WHITE}=3 \\ \text{GREEN}=6 \end{gathered}`

The total number of marbles will be

`7+8+2+3+6=26`

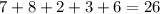
1) To calculate the probability of selecting a red marble replacing it and then selecting a green marble

`\begin{gathered} Pr(\text{red)}=\frac{Number\text{ of red}}{\text{Total number of marbles}} \\ Pr(\text{red)}=(7)/(26) \\ Pr(\text{green)}=\frac{Number\text{ of gr}een}{\text{total number of marbles}} \\ Pr(\text{green)}=(6)/(26)(\text{with replacement)} \\ \text{therefore}, \\ Pr(\text{red and gre}en)=Pr(red)* Pr(green) \\ Pr(\text{red and gre}en)=(7)/(26)*(6)/(26) \\ Pr(\text{red and gr}een)=(21)/(338) \end{gathered}`

Hence,

The probability of selecting a red marble replacing it and then selecting a

green marble is 21/338

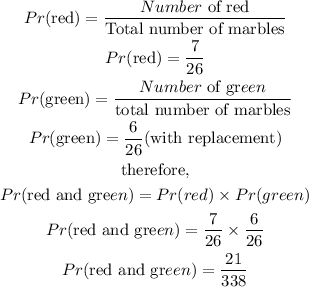
2) To calculate the probability of selecting a blue marble replacing it and then selecting another blue marble

`\begin{gathered} Pr(blue\text{)}=\frac{Number\text{ of blue}}{\text{Total number of marbles}} \\ Pr(\text{blue)}=(8)/(26) \\ \text{with replacement the probabilty of picking a second blue marble will be the}8 \\ Pr(\text{second blue)=}(8)/(26) \\ Pr(\text{blue and blue)=}(8)/(26)*(8)/(26) \\ Pr(\text{blue and blue)=}(16)/(169) \end{gathered}`

Hence,

The probability of selecting a blue marble replacing it and then selecting another blue marble is 16/169

3) To calculate the probability of selecting a white marble and then selecting a green marble without replacing the first marble

`\begin{gathered} Pr(white\text{)}=\frac{Number\text{ of white}}{\text{Total number of marbles}} \\ Pr(\text{white)}=(3)/(26) \\ \text{without replacement the toal number of marbles reduces from 26 to 25} \\ \text{therefore,} \\ Pr(\text{green)}=\frac{Number\text{ of green}}{\text{Total number of remaining marbles}} \\ Pr(\text{green)}=(6)/(25) \\ \text{Hence,} \\ Pr(\text{white and gre}en\text{ without replacement)=}(3)/(26)*(6)/(25)=(9)/(325) \end{gathered}`

Hence,

The probability of selecting a white marble and then selecting a green marble without replacing the first is 9/325

4) To calculate the probability of selecting a red marble and then selecting another red marble without replacing the first marble

`\begin{gathered} Pr(\text{red)}=\frac{Number\text{ of red}}{\text{Total number of marbles}} \\ Pr(\text{red)}=(7)/(26) \\ \text{without replacement the toal number of marbles reduces from 26 to 25} \\ \text{While the number of red reduces from 7 to 6. } \\ \text{therefore,} \\ Pr(\text{another red without replcaement)=}(6)/(25) \\ Pr(\text{red and red without replacement)=}(7)/(26)*(6)/(25)=(21)/(325) \end{gathered}`

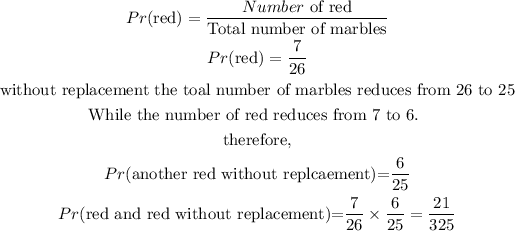
Hence,

The probability of selecting a red marble and then selecting another red marble without replacing the first marble is 21/325