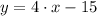Recall that the point-slope form of a line is of the form y=mx+b. We are told that the point (4,1) is on our line and that the slope is 4. This means that m=4. So far, we have the equation
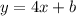
So it only suffices to find the value of b.
Since (4,1) is on this line, it should happen that if we replace x by 4, it must happen that y is 1.
Then, we have the following
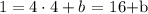
So, if we subtract 15 on both sides, we get
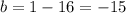
Then the equation of the line is