Question

A committee must be formed with 5 teachers and 3 students. If there are 8 teachers to choose from, and 10 students, how many different ways could the committee be made?

Answer 1

Answer:

The committee could be made in 6,720 ways

Step-by-step explanation:

Parameters:

• Total number of teachers avalable = 8

• Number of teachers to be chosen from this = 5

• Total number of students = 10

• Number of students to be chosen from this = 3

We have number of ways for each group to be:

Teachers:

`\begin{gathered} 8C5=(8!)/((8-5)!5!) \\ \\ =(8!)/(3!5!)=(8*7*6)/(3*2*1)=56 \end{gathered}`

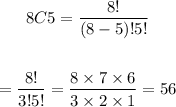
Students:

`\begin{gathered} 10C3=(10!)/((10-3)!3!) \\ \\ =(10!)/(7!3!)=(10*9*8)/(3*2*1)=120 \end{gathered}`

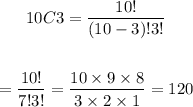
Finally, the committee could be made in

`120*56=6,720ways`