Question

in two or more sentences, describe the transformation(s) that take place on the parent function f(x) = 3^x to obtain the graph f(x) = 3^x-1 - 2

Answer 1

`\begin{gathered} \text{Translation in x}\rightarrow1\text{ right} \\ \text{Translation in y}\rightarrow1\text{ Down} \end{gathered}`

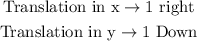
Step-by-step explanation: Certain transformation on the following functions are applied to obtain a new function, we need to find the transformation that was applied on the original function:

Original Function:

`f(x)=3^x`

New function:

`f(x)=3^(x-1)-1^{}`

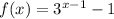
Graph of the two look like:

From this, we see that the original function is in red and the new is in blue, the new function is moved +1 in x (right) and 1 unit in y (down), therefore the transformation is along x-axis and y-axis only!