Question

write an equation of the line in the point- slope form that passes through the given points in the table. Then write the equation in slope-intercept form. (10,80) (5,65)

Answer 1

To write the equation of the line that passes through (10,80) (5,65)

we will use the formula;

`y-y_1\text{ =}(y_(2-)y_1)/(x_2-x_1)(x-x_1)`

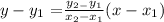
x₁ = 10 y₁ = 80 x₂ = 5 y₂ = 65

substituting the above into the formula;

`y\text{ - 80 = }\frac{65\text{ - 80}}{5\text{ - 10}}(\text{ x - 10)}`

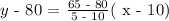
we will go ahead and simplify

`y\text{ - 80 = }(-15)/(-5)(x-10)`

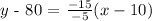
y- 80 = 3(x - 10)

y- 80 = 3x - 30

y -3x -80 + 30 = 0

y - 3x - 50 = 0

The above is the equation of the line.

To write in a slope-intercept form simply means to write it in the form;

y = mx + b

where m is the slope and b is the intercept

Hence;

y = 3x + 50

The above is the equation of the line in a slope-intercept form